東大理科第5問解答
(1) 複素数平面の図形を,原点のまわりに $ -\arg(z) $ 回転し, $ -1 $ 平行移動し, $ \dfrac{\pi}{2} $ 回転すると, 点Pにおける円 $ C $ の接線が実軸に移る. このとき,1と $ u $ はそれぞれ \begin{eqnarray*} &&1\to \dfrac{1}{z}\to \dfrac{1}{z}-1\to i\left(\dfrac{1}{z}-1 \right)\\ &&u\to \dfrac{u}{z}\to \dfrac{u}{z}-1\to i\left(\dfrac{u}{z}-1 \right) \end{eqnarray*} と移る.そして得られた2点は互いに共役である.よって \[ -i\left(\dfrac{1}{\bar{z}}-1 \right)=i\left(\dfrac{u}{z}-1 \right) \] $ z\bar{z}=1 $ を用いて $ u $ について解くと, \[ u=2z-z^2 \] また, \begin{eqnarray*} \dfrac{\bar{w}}{w}&=&\dfrac{1-u}{1-\bar{u}}=\dfrac{1-2z+z^2}{1-2\bar{z}+\bar{z}^2}\\ &=&\left(\dfrac{1-z}{1-\bar{z}} \right)^2=\left(\dfrac{1-z}{z\bar{z}-\bar{z}} \right)^2 =\left(-\dfrac{1}{\bar{z}}\right)^2=z^2 \end{eqnarray*} さらに, \begin{eqnarray*} \dfrac{|w+\bar{w}-1|}{|w|}&=&\left|1+\dfrac{\bar{w}}{w}-\dfrac{1}{w} \right| =\left|1+z^2-1+(2z-z^2)\right|=|2z|=2 \end{eqnarray*} である.
(2)
$ w=X+iY $ とおく.(1)より
\[
2|w|=|w+\bar{w}-1|
\]
なので,
\[
2\sqrt{X^2+Y^2}=|2X-1|
\]
非負の両辺を2乗して
\[
4(X^2+Y^2)=(2X-1)^2
\]
である.これから
\[
4Y^2=-4X+1
\]
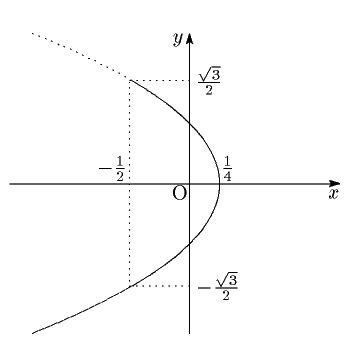
つまり,点Qは放物線 $ 4x+4y^2=1 $ 上を動く.
その範囲を求める.
$ z $ は $ C $ 上にある実部が $ \dfrac{1}{2} $ 以下の複素数なので,
$ z=\cos\theta+i\sin\theta\ \left(\dfrac{\pi}{3}\leqq \theta\leqq \dfrac{5\pi}{3} \right) $
とおける.このとき,
\begin{eqnarray*}
1-z&=&1-\cos\theta-i\sin\theta=2\sin^2\dfrac{\theta}{2}-2i\sin\dfrac{\theta}{2}\cos\dfrac{\theta}{2}\\
&=&2\sin\dfrac{\theta}{2}\left(\sin\dfrac{\theta}{2}-i\cos\dfrac{\theta}{2} \right)\\
&=&2\sin\dfrac{\theta}{2}\left(\cos\dfrac{\theta-\pi}{2}+i\sin\dfrac{\theta-\pi}{2}\right)
\end{eqnarray*}
ここで,
\[
-\dfrac{\pi}{3}\leqq \dfrac{\theta-\pi}{2}\leqq \dfrac{\pi}{3}
\]
であるが, $ \dfrac{\pi}{3}\leqq \theta\leqq \dfrac{5\pi}{3} $ のとき, $ \sin\dfrac{\theta}{2}>0 $ なので,
\[
-\dfrac{\pi}{3}\leqq \arg(1-z)\leqq \dfrac{\pi}{3}
\]
この結果,
\[
\arg w=\arg \dfrac{1}{(1-z)^2}=-2\arg (1-z)
\]
より,
\[
-\dfrac{2\pi}{3}\leqq \arg w \leqq \dfrac{2\pi}{3}
\]
$ \arg w =\pm \dfrac{2\pi}{3} $ となるのは,
点 $ \mathrm{Q}(w) $ が放物線 $ 4x+4y^2=1 $ と直線 $ y=\pm \sqrt{3} $ の交点のうち,
実数部分が負の点になるときである. $ y $ を消去して
\[
12x^2+4x-1=(2x+1)(6x-1)
\]
より, $ x=-\dfrac{1}{2} $ , $ y=\pm \dfrac{\sqrt{3}}{2} $
のときである.